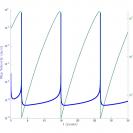
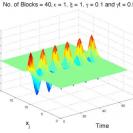
The research develops a new mathematical theory of stochastic nonlinear partial differential equations to understand and advance the statistical theory of earthquakes. New models that give a better description of earthquake faults will be found and special solutions (solitons and breathers) that represent localized earthquakes propagating along the faults are studied. The mathematical seismology gives a firm foundation for the analysis of earthquake dynamics.
The goal is to understand and predict the ground accelerations in large earthquakes. Recent earthquakes in Japan, Taiwan, and the United States have provided ground motions that are quite a bit larger than anticipated from the commonly used ground motion prediction equations. Since the ground motion is the main cause of damage and deaths in an earthquake the research may lead to better preparedness, less damage and fewer deaths in large earthquakes whose timing unfortunately is unpredictable.